Blender Pythonでポリゴンの法線をグローバル座標系で取得して表示する

import bpy
import bmesh
import mathutils
import numpy
import math

# @brief オブジェクトの指定したpolygonの法線を取得
# @param [in] obj オブジェクト
# @param [in] polyIndex ポリゴン番号
# @return 法線（グローバル座標系）
def got_polygon_normal(obj,polyIndex):
    # オブジェクトの行列を取得
    local44 = obj.matrix_world
    
    ll_inv = local44.inverted()
    ll_inv_norm = ll_inv.transposed().to_3x3()    
    
    
    vn = obj.data.polygons[polyIndex].normal
    
    return ll_inv_norm @ vn

# @brief 任意軸回転
# @sa https://www.study.suzulang.com/bpy/bpy-arbitrary-axis-rotation
# @param [in,out] obj 回転するオブジェクト
# @param [in] axis 回転軸
# @param [in] radian 回転角をラジアンで指定
# @return なし
def rotate_object(obj,axis,radian):
    
    rot_mat= mathutils.Matrix.Rotation( radian, 4, axis ) 
    
    # decompose world_matrix's components, and from them assemble 4x4 matrices
    orig_loc, orig_rot, orig_scale = obj.matrix_world.decompose()
    #
    orig_loc_mat   = mathutils.Matrix.Translation(orig_loc)
    orig_rot_mat   = orig_rot.to_matrix().to_4x4()
    orig_scale_mat = (mathutils.Matrix.Scale(orig_scale[0],4,(1,0,0)) @ 
                      mathutils.Matrix.Scale(orig_scale[1],4,(0,1,0)) @ 
                      mathutils.Matrix.Scale(orig_scale[2],4,(0,0,1)))
    #
    # assemble the new matrix
    obj.matrix_world = orig_loc_mat @ rot_mat @ orig_rot_mat @ orig_scale_mat 

# @brief (0,0,1)のベクトルを法線方向に回転するための回転軸と回転角度を求める
# @param [in] the_normal 今回使用する法線
# @return 回転軸,回転角(ラジアン)
def calc_to_normal(the_normal):
    
    Zvec = mathutils.Vector((0,0,1))
    vn = the_normal
    
    onorm = mathutils.Vector(vn)
    onorm.normalize()
    
    
    # 外積
    axis = mathutils.Vector.cross(Zvec,onorm)
    axis.normalize()
    
    # 内積
    th = mathutils.Vector.dot(Zvec,onorm)
    
    # 角度算出
    rad = math.acos( numpy.clip(th,-1.0,1.0) )

    return axis , rad

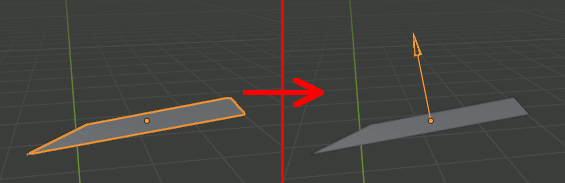
# @brief オブジェクトの０番目のポリゴンの法線を計算し、empty arrowで表示する
# @param [in] target 目的のオブジェクト
def disp_polygon_normal(target):

    tnormal = got_polygon_normal(target,0)

    axis , rad = calc_to_normal(tnormal)


    bpy.ops.object.empty_add(type="SINGLE_ARROW")
    arrow = bpy.context.active_object

    rotate_object(arrow,axis,rad)

    arrow.location = target.location

# 実行例
target = bpy.context.active_object
disp_polygon_normal(target)