月火水木金土日
gluLookAtの自前実装
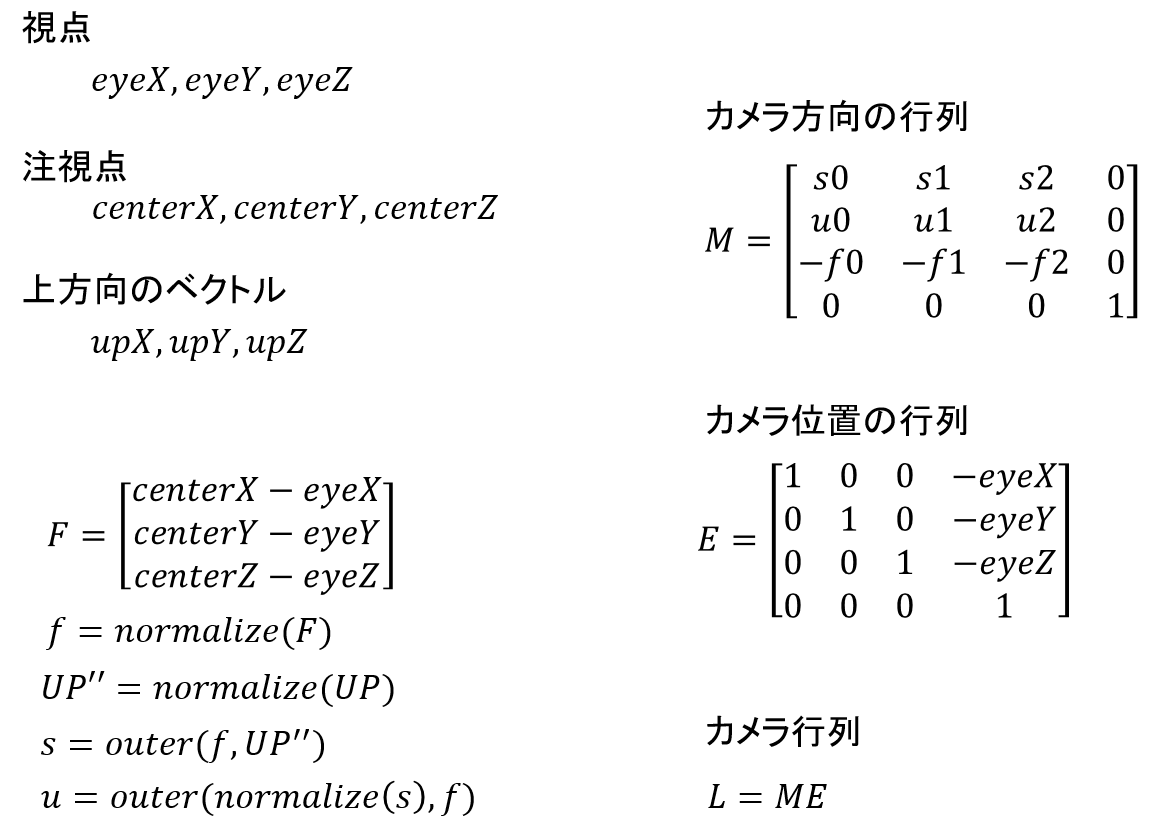
以下の式をそのまま実装する。
https://www.khronos.org/registry/OpenGL-Refpages/gl2.1/xhtml/gluLookAt.xml
プログラム本体
#include <gl/GLU.h> #pragma comment(lib,"glu32.lib") #pragma warning(disable:4996)
//! @brief IxJ行列の i,j の要素にアクセスする //! @param [in] i 行番号 //! @param [in] j 列番号 //! @param [in] I 行数 inline int matijI(const int i, const int j, const int I) { return i + I * j; }
//! @brief IxJ行列 × JxK行列 の乗算関数 //https://suzulang.com/cpp-multmatrix44/ //! @param [out] C Cik 計算結果格納先 //! @param [in] A Aijの行列 //! @param [in] B Bjkの行列 //! @param [in] I Aの行列の行数 //! @param [in] J Aの行列の列数 //! @param [in] K Bの行列の列数 //! @return C template< typename real_tC, typename real_tA, typename real_tB> real_tC* mult_matrixIJK(real_tC* C, const real_tA* A, const real_tB* B, const int I, const int J, const int K) { for (int i = 0; i < I; i++) for (int k = 0; k < K; k++) { C[matijI(i, k, I)] = 0.0; for (int j = 0; j < J; j++) { C[matijI(i, k, I)] += A[matijI(i, j, I)] * B[matijI(j, k, J)]; } } return C; }
//! @brief 4x4行列の行列の積を計算する //! @param [out] C 結果の格納先 //! @param [in] A 行列A //! @param [in] B 行列B //! @return Cへのポインタ template< typename real_tC, typename real_tA, typename real_tB> real_tC* mult_matrix44( real_tC* C, const real_tA* A, const real_tB* B){ return mult_matrixIJK(C, A, B, 4, 4, 4); }
//! @brief 移動行列を作成する //! @param [out] m 結果の格納先 //! @param [in] x ベクトルのx成分 //! @param [in] y ベクトルのy成分 //! @param [in] z ベクトルのz成分 //! @return なし template<typename real_t> void mytranslate( real_t* m, real_t x, real_t y, real_t z ) { m[0] = 1.0; m[1] = 0.0; m[2] = 0.0; m[3] = 0.0; m[4] = 0.0; m[5] = 1.0; m[6] = 0.0; m[7] = 0.0; m[8] = 0.0; m[9] = 0.0; m[10] = 1.0; m[11] = 0.0; m[12] = x; m[13] = y; m[14] = z; m[15] = 1.0; }
//! @brief 単位行列を作成する //! @param [out] m 結果の格納先 //! @return なし template<typename real_t> inline void loadidentity3(real_t* m){ m[0] = 1.0; m[1] = 0.0; m[2] = 0.0; m[3] = 0.0; m[4] = 0.0; m[5] = 1.0; m[6] = 0.0; m[7] = 0.0; m[8] = 0.0; m[9] = 0.0; m[10] = 1.0; m[11] = 0.0; m[12] = 0; m[13] = 0; m[14] = 0; m[15] = 1.0; }
//! @brief 配列にNANが入っているか確認 //! @param [in] m 評価する配列 //! @param [in] count 行列の要素数 template<typename real_t> bool chek_array_nan(const real_t* m, const size_t count) { for (size_t i = 0; i < count; i++) { if (isnan(m[i]) == true) return true; } return false; }
template<typename real_t> inline void Outer3(real_t* const dvec, real_t const* const svec1, real_t const* const svec2) { const real_t& x1 = svec1[0]; const real_t& y1 = svec1[1]; const real_t& z1 = svec1[2]; const real_t& x2 = svec2[0]; const real_t& y2 = svec2[1]; const real_t& z2 = svec2[2]; dvec[0] = static_cast<real_t>(y1 * z2 - z1 * y2); dvec[1] = static_cast<real_t>(z1 * x2 - x1 * z2); dvec[2] = static_cast<real_t>(x1 * y2 - y1 * x2); }
template<typename real_t> real_t Inner3(real_t const* const vec1, real_t const* const vec2) { const int X = 0; const int Y = 1; const int Z = 2; return ((vec1[X]) * (vec2[X]) + (vec1[Y]) * (vec2[Y]) + (vec1[Z]) * (vec2[Z])); }
template<typename real_t> real_t Length3(const real_t* const vec) { const int X = 0; const int Y = 1; const int Z = 2; return sqrt(vec[X] * vec[X] + vec[Y] * vec[Y] + vec[Z] * vec[Z]); }
template<typename real_t> bool Normalize3(real_t * const pV) { const int X = 0; const int Y = 1; const int Z = 2; real_t len; real_t& x = pV[X]; real_t& y = pV[Y]; real_t& z = pV[Z]; len = static_cast<real_t>(sqrt(x * x + y * y + z * z)); if (len < static_cast<real_t>(1e-6)) return false; len = static_cast<real_t>(1.0) / len; x *= len; y *= len; z *= len; return true; }
//! @brief gluLookAtの自前実装版 //! @param [out] M 結果の格納先 //! @param [out] eyeX カメラ位置 //! @param [out] eyeY カメラ位置 //! @param [out] eyeZ カメラ位置 //! @param [out] centerX //! @param [out] centerY //! @param [out] centerZ //! @param [out] upX //! @param [out] upY //! @param [out] upZ //! @return なし
void myglulookat( GLdouble* M, GLdouble eyeX, GLdouble eyeY, GLdouble eyeZ, GLdouble centerX, GLdouble centerY, GLdouble centerZ, GLdouble upX, GLdouble upY, GLdouble upZ) { GLdouble f[3] = { centerX - eyeX, centerY - eyeY, centerZ - eyeZ }; Normalize3(f); GLdouble UP[3] = {upX,upY,upZ}; Normalize3(UP); GLdouble s[3]; Outer3(s, f, UP); Normalize3(s); GLdouble u[3]; Outer3(u, s, f); GLdouble M0[16]; M0[ 0] = s[0]; M0[ 1] = u[0]; M0[ 2] = -f[0]; M0[ 3] = 0; M0[ 4] = s[1]; M0[ 5] = u[1]; M0[ 6] = -f[1]; M0[ 7] = 0; M0[ 8] = s[2]; M0[ 9] = u[2]; M0[10] = -f[2]; M0[11] = 0; M0[12] = 0; M0[13] = 0; M0[14] = 0; M0[15] = 1; ///////////////////////////// ///////////////////////////// GLdouble E[16]; mytranslate(E, -eyeX, -eyeY, -eyeZ); mult_matrix44(M, M0, E); if (chek_array_nan(M, 16) == true) loadidentity3(M); }
テスト用コード
void test() { glMatrixMode(GL_PROJECTION); glLoadIdentity(); glMatrixMode(GL_MODELVIEW); glLoadIdentity(); GLdouble ex = (rand() % 1000) / 1000.0 - 0.5; GLdouble ey = (rand() % 1000) / 1000.0 - 0.5; GLdouble ez = (rand() % 1000) / 1000.0 - 0.5; GLdouble cx = (rand() % 1000) / 1000.0 - 0.5; GLdouble cy = (rand() % 1000) / 1000.0 - 0.5; GLdouble cz = (rand() % 1000) / 1000.0 - 0.5; GLdouble ux = (rand() % 1000) / 1000.0 - 0.5; GLdouble uy = (rand() % 1000) / 1000.0 - 0.5; GLdouble uz = (rand() % 1000) / 1000.0 - 0.5;
GLdouble M0[16]; gluLookAt(ex, ey, ez, cx, cy, cz, ux, uy, uz); glGetDoublev(GL_MODELVIEW_MATRIX, M0);
GLdouble M1[16]; myglulookat( M1, ex, ey, ez, cx, cy, cz, ux, uy, uz);
puts("------------------------"); for (size_t i = 0; i < 16; i++) { printf("[%d] %lf\n", i, M0[i] - M1[i]); } }
実行結果
[0] -0.000000 [1] 0.000000 [2] -0.000000 [3] 0.000000 [4] -0.000000 [5] 0.000000 [6] -0.000000 [7] 0.000000 [8] -0.000000 [9] -0.000000 [10] 0.000000 [11] 0.000000 [12] 0.000000 [13] 0.000000 [14] -0.000000 [15] 0.000000